Aufgabe:
F(x), Funktion, Log Log Plot
Problem/Ansatz:
Ich habe einen Gas Sensor und möchte den PPM wert ermitteln.
Hier die webseite dazu. https://www.teachmemicro.com/use-mq-7-carbon-monoxide-sensor/
Es ist soweit alles verständlich aber ich brauche Hilfe bei der letzen Zeile.
ppm = (1538,46\( \frac{RS}{R0} \))-1.709
Wie kommt man auf die 1538,46 ??
Lg Sebastian
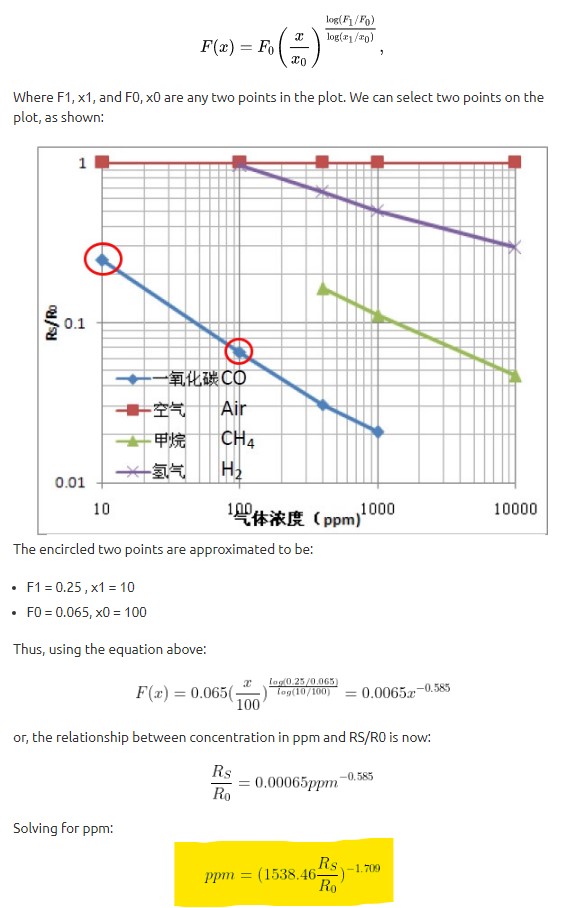
Text erkannt:
\( F(x)=F_{0}\left(\frac{x}{x_{0}}\right)^{\frac{\log \left(F_{1} / F_{0}\right)}{\log \left(x_{1} / x_{0}\right)}} \)
Where \( F 1, x 1 \), and F0, \( x 0 \) are any two points in the plot. We can select two points on the plot, as shown:
The encircled two points are approximated to be:
- \( F 1=0.25, x 1=10 \)
- \( F 0=0.065, x 0=100 \)
Thus, using the equation above:
\( F(x)=0.065\left(\frac{x}{100}\right)^{\frac{\operatorname{logog}(0.25 / 0.065)}{\log (10 / 100)}}=0.0065 x^{-0.585} \)
or, the relationship between concentration in \( \mathrm{ppm} \) and \( \mathrm{RS} / \mathrm{RO} \) is now:
\( \frac{R_{S}}{R_{0}}=0.00065 \mathrm{ppm}^{-0.585} \)
Solving for \( \mathrm{ppm} \) :
\( p p m=\left(1538.46 \frac{R_{S}}{R_{0}}\right)^{-1.709} \)