Ist lim n-> unendlich n-nq+1-q = 1-q... richtig?
hab das folgende Problem:
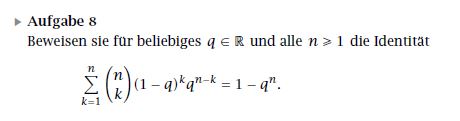
mit der vollständigen Induktion folgt...
.
.
.
↔ $$1-{ q }^{ n }+(n-nq+1-q)\cdot { q }^{ n }=1-{ q }^{ n }\cdot { q }^{ 1 }$$
↔ $$\lim _{ n\rightarrow \infty} 1-1+n-nq+1-q=1-1\cdot { q }$$
da n -> ∞ sind die ns alle 0 und dann folgt
↔ 1 - q = 1 - q
↔ 1= 1 also ist der beweis fertig?